NCERT Exemplar for Class 11 Physics - Law of Motion - Free PDF Download
Free PDF download of NCERT Exemplar for Class 11 Physics Chapter 5 - Law of Motion solved by expert Physics teachers on Vedantu.com as per NCERT (CBSE) Book guidelines. All Chapter 5 - Law of Motion exercise questions with solutions to help you to revise the complete syllabus and score more marks in your examinations.
Access NCERT Exemplar Solutions for Class 11 Physics Chapter 5 - Laws of Motion
Exercise
MULTIPLE CHOICE QUESTIONS (TYPE-I)
5.1. A ball is travelling with uniform translatory motion. This means that:
(a) It is at rest.
(b) The path can be a straight line or circular and the ball travels with uniform speed.
(c) All particles of the ball have the same velocity (magnitude and direction) and the velocity is constant.
(d) The centre of mass the ball moves with constant velocity and the ball spins about its centre uniformly.
Ans: Option (c)
Uniform translatory motion occurs when all the particles of the body move with the same velocity in the same straight line. Hence, the correct answer is (c).
5.2. A meter scale is moving with uniform velocity. This implies
(a) The net acting on the scale is zero, but a torque about the centre of mass can act on the scale.
(b) The net force acting on the scale is zero, and the net torque acting about the centre of mass of the scale is also zero.
(c) The net force acting on it need not be zero but the torque on it is zero.
(d) Neither the force nor the torque needs to be zero.
Ans: Option (b)
Since the body is moving with a uniform velocity, hence its acceleration is zero. This implies that the net force acting on it must be zero, which further makes the torque acting about the centre of mass of the scale also zero. Hence, the correct answer is (b).
5.3. A cricket ball of mass 150 g has the initial velocity
(a) zero
(b)
(c)
(d)
Ans: Option (c)
Since momentum is given by
Hence,
That is,
Hence, the correct answer is (c).
5.4. In a previous problem 5.3, the magnitude of momentum transferred during the hit is:
(a) zero
(b)
(c)
(d)
Ans: Option (c)
Since we have
Therefore, the magnitude is
Hence, the correct answer is (c).
5.5. Conservation of momentum is a collision between particles can be understood from
(a) Conservation of energy
(b) Newton’s first law only
(c) Newton’s second law only
(d) Both Newton’s second and third law
Ans: Option (d)
By the Newton’s second law of motion we can write
Since, by the law of conservation of momentum we have
Therefore,
Hence momentum is constant.
Also by the Newton’s third law of motion, we have
That gives
Here the law of conservation of momentum is proved.
Hence, the correct answer is (d).
5.6. A hockey player is moving northward and suddenly turns westward with the same speed to avoid an opponent. The force that acts on the player is:
(a) frictional force along westward
(b) muscle force along southward
(c) friction force along the south-west
(d) muscle force acting south-west
Ans: Option (c)
Since the frictional force always tries to resist the motion, hence the force that acts on the player moving northward and then suddenly turning westward is the frictional force acting along the south-west direction. Hence, the correct answer is (c).
5.7. A body of mass 2kg travels according to the law
(a) 136 N
(b) 134 N
(c) 158 N
(d) 68 N
Ans: Option (a)
Given that
Hence,
Now,
And
Now when
Then
Hence, the correct answer is (a).
5.8. A body of mass 5 kg is acted upon by a force
(a) never
(b) 10 s
(c) 2s
(d) 15s
Ans: Option (b)
Since
Now, since the x-component of the final velocity is zero,
Hence,
That gives us
Hence, the correct answer is (b).
5.9. A car of mass m starts from rest and acquires a velocity
(a)
(b)
(c) more than
(d)
Ans: Option (b)
Since we know that
Here
Therefore,
Hence,
Now,
Therefore, the force
Hence, the correct answer is (b).
MULTIPLE CHOICE QUESTIONS-II
5.10. The motion of a particle of mass m is given by
(a) The force at
(b) The particle is acted upon by on impulse of magnitude
(c) The particle is not acted upon by any force.
(d) The particle is not acted upon by a constant force.
(e) There is no impulse acting on the particle
Ans: Options (a), (b) and (d)
Since the force acting on the particle is a function of time, hence it is not a constant force.
Now at
That is
Now, since impulse is the change in momentum given by
Hence,
Hence, the correct answers are (a), (b) and (d).
5.11. In the given figure, the coefficient of friction between the floor and the body B is 0.1. The coefficient of friction between bodies B and A is 0.2. A force F is applied as shown on B. the mass of A is m/2 and of B is m. Which of the following statements are true?

(a) The bodies will move together if
(b) The body A will slip with respect to B if
(c) The bodies will move together if
(d) The bodies will be at rest if
(e) The maximum value of
Ans: Options (a), (b) (d) and (e)
Let the frictional force acting in the opposite directions on the bodies A and B be
Now the common acceleration of bodies A and B will be given by
Hence the force on A is
Now if
Hence,
So,
Also,
Hence we can write
Or,
Now the minimum force which can move A and B together is
That is
Hence,
Therefore only the option (c) is incorrect.
Hence, the correct answers are (a), (b), (d) and (e).
5.12. Mass
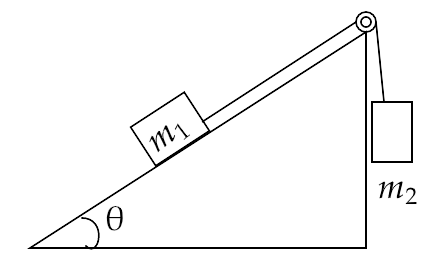
(a) If
(b) If
(c) If
(d) If
Ans: Options (b) and (d)
The system can be represented by the figure given below.
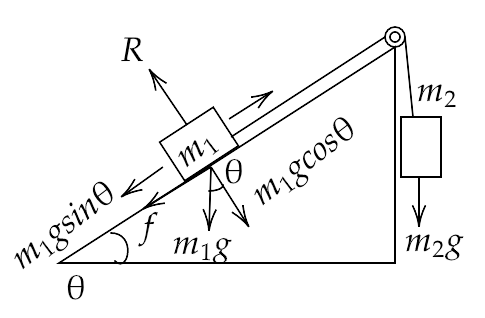
Here, we can write
Now
That gives
Or,
Hence,
Also,
That gives
Or,
Hence,
Therefore, the correct answers are (b) and (d).
5.13. In a given figure, a body A of mass m slides on a plane inclined at an angle
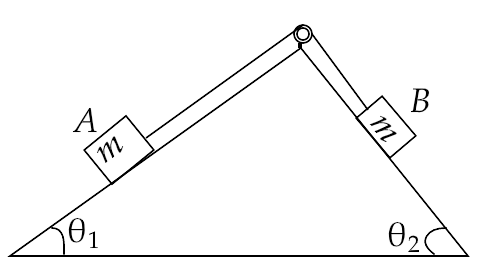
(a) A will never move up the plane.
(b) A will just start moving up the plane when
(c) For A to move up the plane,
(d) B will always slide down with constant speed.
Ans: Options (b) and (c)
This system can be represented by the figure given below.
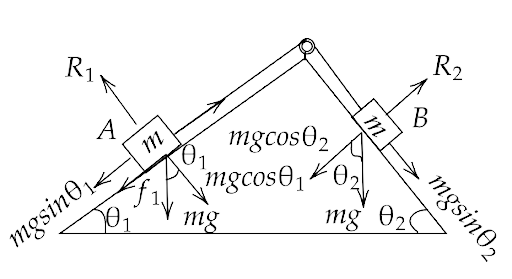
Here we have
Now, the body A will move up and body B will move down the plane,
If
That gives us,
Or,
Hence,
Also, the body A will move down and body B will move up the plane,
If
Or,
Hence,
Hence, the correct answers are (b) and (c).
5.14. Two billiard balls A and B, each of mass 50g and moving in opposite directions with speed of 5m/s each, collide and rebound with the same speed. If the collision lasts for
(a) The impulse imparted to each ball is 0.25 kg m/s and the force on each ball is 250 N.
(b) The impulse imparted to each ball is 0.25 kg m/s and the force exerted on each ball is
(c) The impulse imparted to each ball is 0.5 Ns.
(d) The impulse and the force on each ball are equal in magnitude and opposite in direction.
Ans: Options (c) and (d)
We are given that,
Hence we have initial momentum of each ball
After collision, both balls will move in opposite directions
Hence, we have final momentum of each ball
Now the impulse is the change in momentum,
Hence,
Therefore, the correct answers are (c) and (d).
5.15. A body of mass 10kg is acted upon by two perpendicular forces, 6 N and 8 N. The resultant acceleration of the body is
(a)
(b)
(c)
(d)
Ans: Options (a) and (c)
Since the two forces are acting perpendicular, hence their resultant force will be given by,
Hence the acceleration will be
Now we have
And
Therefore, the correct answers are (a) and (c).
VERY SHORT ANSWER TYPE QUESTIONS
5.16. A girl riding a bicycle along a straight road at a speed of
Ans: Given that,
Also their initial velocities are
And their final velocities are
Now according to the law of conservation of momentum, we have the total initial momentum equal to the total final momentum.
Therefore,
That gives,
Hence,
Therefore the change in speed is
5.17. A person of mass 50 kg stands on a weighing scale on a lift. If the lift is descending with a downward acceleration of
Ans: Since the lift is moving downwards, hence it will result in a decrease in the weight of the person.
The new weight of the person inside the lift will be given by
Hence,
Now the reading of the weighing scale will be
5.18. The position-time graph of a body of mass 2 kg is as given in Figure. What is the impulse on the body at t= 0s and t= 4s?
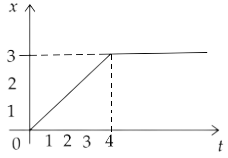
Ans: From the figure, we can see that the graph is a straight line between t= 0s and t= 4s , hence the velocity is constant in this period.
The final velocity will be given by the slope of the graph,
Hence
Since the velocity is zero at t= 0s and t= 4s ,hence the momentum is also zero at these two points.
Now since impulse is change in momentum,
Hence, the impulse at t= 0s is
Similarly, the impulse at t= 4s is
Therefore, the impulse first increased at t= 0s by
5.19. A person driving a car suddenly applies the brakes on seeing a child on the road ahead. If he is not wearing the seat belt, he falls forward and hits his head against the steering wheel. Why?
Ans: When the car is moving with some velocity, then the person is also moving with the same velocity. After applying the brakes, the car stops suddenly. The lower part of the driver stops along with the car but the upper part of the person continues to move with the existing velocity. This results in the person hitting his head against the steering wheel due to the inertia of motion.
5.20. The velocity of a body of mass 2 kg as a function of t is given by
Ans: Given that
Momentum at t= 2 s will be given by
That gives us,
Since
Also the force acting on it will be given by
Since
5.21. A block placed on a rough horizontal surface is pulled by a horizontal force F. Let f be the force applied by the rough surface on the block. Plot a graph of f versus F.
Ans: For the smaller values of the applied force the frictional force increases linearly upto the limiting friction as shown in the graph below.
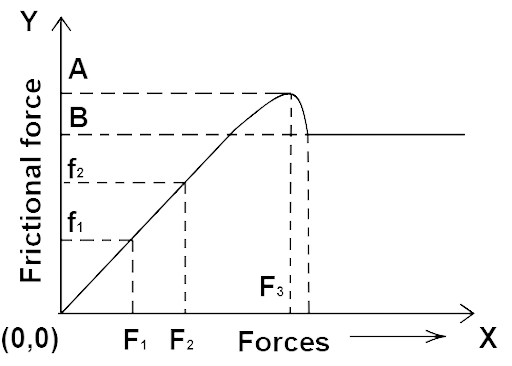
After crossing the maximum point of the static friction the frictional force becomes constant and does not change due to the kinetic friction.
5.22. Why are porcelain objects wrapped in paper or straw before packing for transportation?
Ans: Due to the brittle nature of porcelain objects, they can be broken even after getting a small jerk while transportation. Wrapping objects in paper and straw helps in increasing the time taken to stop or change their velocity instead of a sudden motion or break. Also, decreasing the acceleration
5.23. Why does a child feel more pain when she/he falls down on a hard cement floor than when she/he falls on the soft muddy ground in the garden?
Ans: Since the mass and velocities in both the cases are same, the only way to decrease the amount of force experienced while hitting the ground is to increase the time taken during the contact because we have
A cement floor is hard in nature and takes very little amount of time while hitting it whereas a muddy ground is soft in nature and takes more time during the impact and hence decreases the experienced force.
5.24. A woman throws an object of mass 500 g with speed of
(a) What is the impulse imparted to the objects?
Ans: Given that the mass of the object is
Now the impulse imparted to the object will be given by,
That gives,
(b) If the object hits a wall and rebounds with half the original speed, what is the change in momentum of the object?
Ans: Now if the object bounces back with half the original velocity,
Then we have
Then the change in momentum will be given by,
Hence,
5.25. Why are mountain roads generally made winding upwards rather than going straight up?
Ans: While going upwards on an inclined plane or a mountain which has a slope, the force of friction acting on a vehicle of mass m is given by
SHORT ANSWER TYPE QUESTIONS
5.26. A mass of 2kg is suspended with thread AB (figure). Thread CD of the same type is attached to the other end of 2 kg mass. Lower thread is pulled gradually, harder and harder in the downward direction so as to apply force on AB. Which of the threads will break and why?
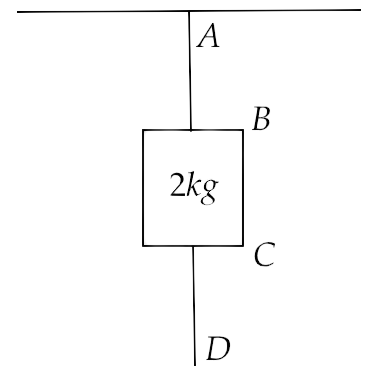
Ans: When the thread CD is pulled downwards then the force acting at point C is equal to the force applied at point D acting downwards. Whereas the force acting at point A is equal to the sum of force applied on CD and the force acting downwards due to the suspended mass of 2kg. Since, the force acting at point A is 2kg more than point C, therefore thread AB will break from the point A.
5.27. In the above-given problem if the lower thread is pulled with a jerk, what happens?
Ans: If thread CD is pulled with a jerk then it will break from the point C because the force acting at point C is not moving further to the point A due to the inertia of the rest of the suspended mass of 2kg.
5.28. Two masses of 5 kg and 3 kg are suspended with help of massless inextensible strings as shown in Figure. Calculate

Ans: Given that, the whole system is going in the upward direction with an acceleration
Also, we have
Now we know that the tension in a string is always equal and opposite in all parts of the string.
Hence, forces acting on mass
That is,
Or,
Hence,
Similarly, forces acting on mass
That is,
Or,
Hence,
Now that gives us
5.29. Block A of weight 100 N rests on a frictionless inclined plane of slope angle 30° (Figure). A flexible cord attached to A passes over a frictionless pulley and is connected to block B of weight W. Find the weight W for which the system is in equilibrium.
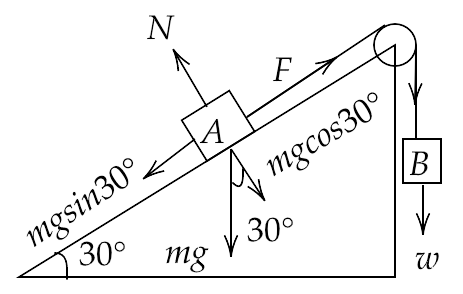
Ans: For the given system to be in an equilibrium, there must be no motion i.e. the frictional force must be equal to zero.
Now, for the balanced condition of A and B, we have
That gives us,
Or,
Since
Therefore,
i.e.
Hence the weight of the block is
5.30. A block of mass M is held against a rough vertical wall by pressing it with a finger. If the coefficient of friction between the block and the wall is μ and the acceleration due to gravity is g, calculate the minimum force required to be applied by the finger to hold the block against the wall?
Ans: Let F be the force applied by the finger on the block of mass M in order to hold it against the wall.
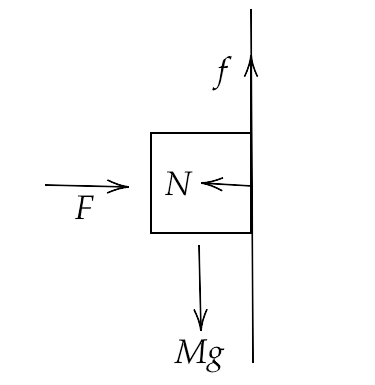
For the equilibrium condition, we have
That gives us,
Hence,
Therefore, the minimum force required to hold the block against the wall at rest is
5.31. A 100kg gun fires a ball of 1kg horizontally from a cliff of height 500m. It falls on the ground at a distance of 400 m from the bottom of the cliff. Find the recoil velocity of the gun. (acceleration due to gravity =
Ans: Let the final velocity of the ball be
Since it is fired horizontally, its vertical component is zero.
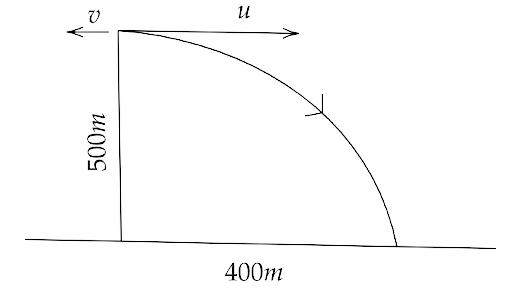
Now for the vertically downward motion of the ball, we have
That gives us,
Or,
Hence,
Now, we can write
Now using the law of conservation of momentum we can write,
That gives us,
Hence,
i.e.
Therefore , the recoil velocity of the gun is
5.32. Figure (a) and (b), shows (x, t), (y, t) diagrams of a particle moving in 2 dimensions If the particle has a mass of 500 g, find the force (direction and magnitude) acting on the particle.
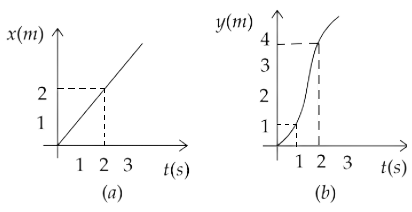
Ans: From the graph (a), we have
Hence,
Since the velocity is constant, hence we have
Therefore,
Now, from the graph (b), we have
Hence,
Now the acceleration will be given by,
Therefore,
Now their resultant force will be given by,
33. A person in an elevator accelerating upwards with an acceleration of
Ans: When an elevator is moving upwards with an acceleration
Now for the effectives motion of the coin we have
Let the time of coin to achieve the maximum height be t
Then we have,
That gives us,
Hence,
So the time to reach the maximum height is
Hence, time taken by the coin to fall back into the hand will be given by,
LONG ANSWER TYPE QUESTIONS
5.34. There are three forces
(a) Show that the forces are coplanar.
Ans: It is given that due to the three forces
Therefore, the acceleration due to these forces is zero.
That gives us the resultant force also equal to zero.
Hence,
This relation states that the force
Therefore if the two forces
Hence the three forces
(b) Show that the torque acting on the body about any point due to these three forces is zero.
Ans: Now, since the resultant of these forces is zero, so the torque acting on the body at any point will also be zero as,
5.35. When a body slides down from rest along a smooth inclined plane making an angle of 45° with the horizontal, it takes time T. When the same body slides down from rest along a rough inclined plane making the same angle and through the same distance, it is seen to take time
Ans: Given that, the body slides down from an inclined plane making an angle of 45° with the horizontal, taking time T.
The effective acceleration of the body in this case will be
Now for this motion we can write,
That gives us,
Or,
Now considering the motion of the body along rough inclined plane, we have
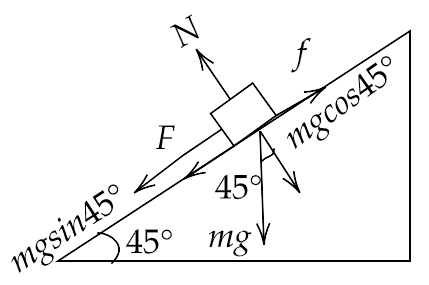
In this case, for the equilibrium condition we can write
That gives,
Or,
Hence,
Now if
Then we have
That gives,
Or,
Now since the distance in both the cases are equal,
Therefore, we have
That gives us,
Or,
Hence,
5.36. Figure (a) and (b) shows
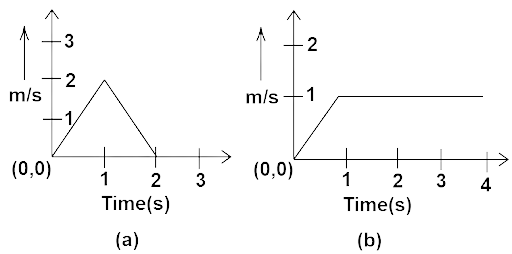
Ans: In the figure (a), we have
Hence the acceleration between 0s to 1s will be given by,
Also
And the acceleration between 1s to 2s will be given by,
Also
Now in figure (b), we have
Hence the acceleration between 0s to 1s will be given by,
Also
And the acceleration between 1s to 3s will be given by,
Also
Now the resultant force will be given by,
Hence,
And,
And,
5.37. A racing car travels on a track (without banking) ABCDEFA (Figure). ABC is a circular arc of radius 2R. CD and FA are straight paths of length R and DEF is a circular arc of radius R = 100 m. The coefficient of friction on the road is μ = 0.1. The maximum speed of the car is
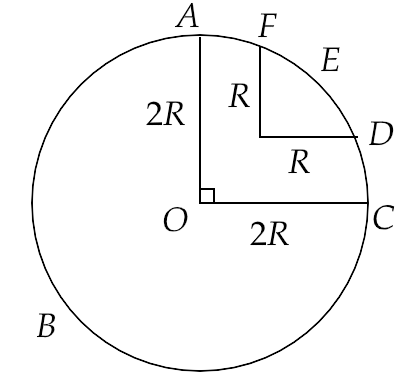
Ans: Since a given circular path is unbanked, hence the necessary centripetal force is provided by the frictional force.
Hence, we have
Or,
Now consider each part of the circular path separately.
For the path ABC, we have
Hence,
That gives,
For the path CD and FA, we have
Since CD and FA are straight path, hence
That gives,
For the path DEF, we have
Hence,
That gives,
Therefore, total time taken by the car to cover the complete distance is,
Hence,
i.e.
5.38. The displacement vector of a particle of mass m is given by
(a) Show that the trajectory is an ellipse.
Ans: Given that
Let
That gives us,
And,
Now squaring and adding these two equations gives us,
Hence,
Therefore the trajectory of the particle is an ellipse.
(b) Show that
Ans: Since
Hence,
And
Also,
Hence,
And,
Therefore, the resultant acceleration will be
That gives us,
Or,
Hence,
Now since,
Therefore,
Hence proved.
5.39. A cricket bowler releases the ball in two different ways:
(a) giving it only horizontal velocity, and
Ans: In case (a),
For horizontal motion, we have
And for vertical motion, we have
Since in this case we are given that
Hence,
So the resultant velocity is
(b) giving it horizontal velocity and a small downward velocity. The speed
Ans: For horizontal motion, we have
And for vertical motion, we have
Hence,
So the resultant velocity is
Comparing these two resultant velocities, we get
Therefore, in case (b) will have a greater speed when the ball hits the ground.
5.40. There are four forces acting at a point P produced by strings as shown in the figure, which is at rest. Find the forces
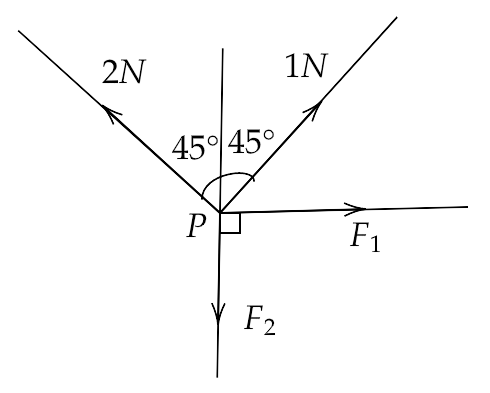
Ans: Since the particle is at rest which means it has no acceleration and the resultant force due to all the forces must be zero. Therefore, the components of the forces along X and Y axes will also be zero. Now, for the equilibrium condition along the X-axis, we have
That gives us,
Hence,
Or,
That is,
Also, for the equilibrium condition along the Y-axis, we have
That gives us,
Or,
Hence,
5.41. A rectangular box lies on a rough inclined surface. The coefficient of friction between the surface and the box is μ. Let the mass of the box be m.
(a) At what angle of inclination θ of the plane to the horizontal will the box just start to slide down the plane?
Ans: The above given system can be represented by the figure drawn below:
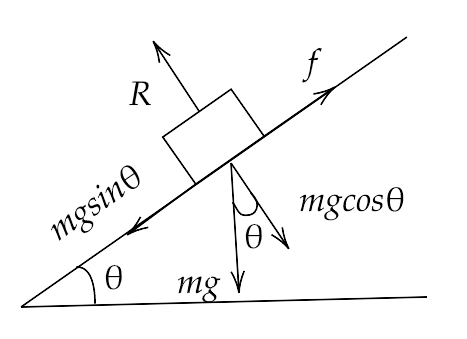
(a) The box will start to slide down the plane if
i.e. when
That gives,
Hence,
(b) What is the force acting on the box down the plane, if the angle of inclination of the plane is increased to α > θ?
Ans: Now if
Hence, we have
Or,
That gives us,
(c) What is the force needed to be applied upwards along the plane to make the box either remain stationary or just move up with uniform speed?
Ans: To keep the box stationary or move upwards with a uniform speed, the net force
must be acting upwards.
Hence, we have
Or,
That gives us,
(d) What is the force needed to be applied upwards along the plane to make the box move up the plane with acceleration a?
Ans: The box will move upwards with an acceleration a if we have,
Or,
That gives us,
Hence,
5.42. A helicopter of mass 2000kg rises with a vertical acceleration of
(a) Force on the floor of the helicopter by the crew and passengers.
Ans: Given that
The force on the floor of the helicopter by the crew and passengers will be given by
That gives us,
Hence,
(b) The action of the rotor of the helicopter on the surrounding air.
Ans: The action of the rotor of the helicopter on the surrounding air will be equal to the reaction force by Newton’s third law.
Hence, we have
That gives us,
Or,
Hence,
(c) Force on the helicopter due to the surrounding air.
Ans: Force on the helicopter due to the surrounding air is the reaction force which is equal and opposite to the action force by the third law of Newton.
Therefore,
i.e.
That gives us,
That is,
Hence,
Or,
Chapter Details
After learning the quantitative description of the motion of a particle in the chapters of 'Motion in a Straight Line' and 'Motion in a Plane,' it's time to learn about the various rules and natural laws governing it. 'Law of Motion' defines the various principles followed by the objects in moving in a three-dimensional space. As the movement of objects depends on various external agencies of the environment, this chapter examines and establishes the laws of inertia and force acting on a body of mass in motion.
You will come to know about any previous philosophers or scientists such as Aristotle belonging to the common era before Christ, in section 5.2 ‘Aristotle's Fallacy,’ Galileo Galilei of the sixteenth and seventeenth century, or Sir Isaac Newton of the seventeenth and eighteenth century. They all are considered the originators of modern-day physics. Newton explained the condition and effect of various forces acting over a body of the motion. The three laws of motion mentioned in sections 5.4, 5.5, and 5.6 given by Newton are universal in nature and it is very important to learn and by heart them. These laws are later used to explain the momentum of the objects in motion and its conservation as well as in equilibrium in sections 5.7 ‘Conservation of Momentum’ and 5.8 ‘Equilibrium of a Particle’. Later the chapter delves us into the more intricate studies about ‘Common Forces of Mechanics’ in section 5.9 such as ‘Friction.’ It includes the sub-topics such as ‘Static Friction,’ and ‘Rolling Friction.’
Section 5.10 about ‘Circular Motion’ has been explained taking an example of a car running around a turning on a levelled road and a banked road. 12 example solutions in this chapter have made it easy to understand. Finally, the chapter has tried to solve the problems in mechanics to make us clear about how to apply the laws while solving the problems in section 5.11 “Solving Problems in Mechanics.’
As said, always solving the problems provided in the exercises is very important for understanding the laws of motion and it's appropriate application. You can use the solved papers of the exercises provided by vedantu.com free of cost. You can download the paper from this page directly and also use it as a reference before solving the problems.
FAQs on NCERT Exemplar for Class 11 Physics Chapter 5 - Law of Motion (Book Solutions)
1. What do I need to learn before learning the ‘laws of motion?
We must have a fair knowledge of the historical events in the development of the natural laws of nature by various philosophers and scientists. Starting with Aristotle in the Greek times and the scientists emerging in the backdrop of the European renaissance of the 16th century. Students are also advised to read about the life history of Sir Isaac Newton.
You are also required to be apt in the Calculus portion of mathematics for using disintegration and integrations for deriving the equations.
2. Should I complete previous chapters before starting the chapter ‘laws of motion?
The previous chapters are very necessary to know about all the basic concepts of motion and its quantification. You can do this from the standard textbooks and get the solutions on our website. After learning all the theories in chapters 1, 2, 3, and 4 it would be easy for you to get a clear picture of the methods used to quantify the laws of the natural world explained in this chapter. So take all your time to complete the previous chapters before going over to the next chapter.
3. Is it necessary to refer to the science textbook of class 9?
Though this chapter deals with the higher concepts of motion, it is always better to have a good hold of the simple explanations of the terms we are going to study. Class 9 Science textbook can be a very good source for this. You can get all the books over the internet and of course, the solutions for class 9 are provided for you on the Vedantu website. It is never a loss of time to be prepared with any available knowledge while starting to learn any new chapter.
4. Can I find all the solutions to the problems of the chapter exercise in Vedantu Book Solutions?
It is always good to solve the problems by yourself after completing the chapter and fully understanding it. Then you can use the solved papers to check your answers and correct the mistakes if any. The questions have been solved by competent experts in the field of physics. It is provided to you without any cost. You can also use these solutions before solving the problems in exercise for a better understanding of the concepts.
5. Can I refer to any other book instead of NCERT Class 11 Physics - Law of Motion ?
Other books available for learning the laws of motion are good for reference but NCERT Class 11 physics book is best for comprehensive understanding and from the examination point of view as well. It is always best to refer to standard materials. The solutions provided by Vedantu are very much accurate and reliable. So you must always go with the Vedantu Class 11 Physics Book solutions. Here you can find all the study and practice materials suitable for your syllabus and exam preparation.























