NCERT Solutions for Class 12 Chemistry In Hindi Chapter 3 Electrochemistry Hindi Medium

1. निम्नलिखित धातुओं को उस क्रम में व्यवस्थित कीजिए जिसमें वे एक-दूसरे को उनके | लवणों के विलयनों में से प्रतिस्थापित करती हैं।

$Al,{\text{ }}Cu,{\text{ }}Fe,{\text{ }}Mg$ एवं $Zn$

उत्तर:  $Mg,{\text{ }}Al,{\text{ }}Zn,{\text{ }}Fe$ तथा $Cu$

2. नीचे दिए गए मानक इलेक्ट्रोड विभवों के आधार पर धातुओं को उनकी बढ़ती हुई अपचायक क्षमता के क्रम में व्यवस्थित कीजिए।

${{K^ + }|K{\text{ }} = {\text{ }} - 2.93{\text{ }}V,{\text{ }}A{g^ + }|{\text{ }}Ag = {\text{ }}0.80V,}$ 

${H{g^{2 + }}|{\text{ }}Hg = {\text{ }}0.79{\text{ }}V}$

${M{g^{2 + }}\left| {{\text{ }}Mg{\text{ }} = {\text{ }} - 2.37{\text{ }}V,{\text{ }}C{r^{3 + }}} \right|{\text{ }}Cr{\text{ }} = {\text{ }} - 0.74{\text{ }}V}$

उत्तर: किसी धातु की अपचायक शक्ति उसके ऑक्सीकरण कार्यक्रम पर निर्भर करती है। ऑक्सीकरण कार्यक्रम जितना अधिक होगा, ऑक्सीकृत होने की प्रवृत्ति  उतनी अधिक होगी और इसलिए उसकी अपचायक शक्ति भी उतनी ही अधिक होगी। अत: दिये गये धातुओं की बढ़ती अपचायक शक्ति का क्रम नीचे दिया गया है–

$Ag{\text{ }} < {\text{ }}Hg{\text{ }} < {\text{ }}Cr{\text{ }} < {\text{ }}Mg{\text{ }} < {\text{ }}K$

3. उस गैल्वेनी सेल को दर्शाइए जिसमें निम्नलिखित अभिक्रिया होती है –

$Zn\left( s \right){\text{ }} + {\text{ }}2A{g^ + }\left( {aq} \right){\text{ }} \to {\text{ }}Z{n^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}2Ag\left( s \right)$

अब बताइए –

• कौन-सा इलेक्ट्रोड ऋणात्मक आवेशित है?

• सेल में विद्युत-धारा के वाहक कौन-से हैं?

• प्रत्येक इलेक्ट्रोड पर होने वाली अभिक्रिया क्या है?

उत्तर: जिंक इलेक्ट्रोड ऐनोड का काम करता है, जबकि सिल्वर इलेक्ट्रोड कैथोड का काम करता है। सेल को निम्न प्रकार प्रदर्शित कर सकते हैं –

$Zn{\text{ }}\left( S \right)\left| {{\text{ }}Z{n^{2 + }}\left( {aq} \right){\text{ }}} \right|\left| {{\text{ }}Ag + {\text{ }}\left( {aq} \right)} \right|{\text{ }}Ag{\text{ }}\left( s \right)$

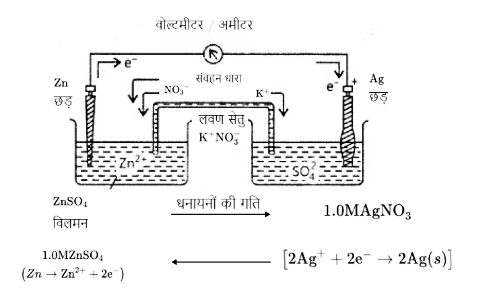
• $Zn{\text{ }}/{\text{ }}Z{n^{2 + }}$ इलेक्ट्रोड ऋणात्मक आवेशित होता है तथा ऐनोड की तरह कार्य करता है।

• बाह्य परिपथ में इलेक्ट्रॉन तथा आंतरिक परिपथ में आयन।

• ऐनोड पर : $Zn{\text{ }}\left( s \right){\text{ }} \to {\text{ }}Z{n^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}2{e^ - }$

• कैथोड पर : $Ag + {\text{ }}\left( {aq} \right){\text{ }} + {\text{ }}{e^ - }^{} \to {\text{ }}Ag\left( s \right)$

4.निम्नलिखित अभिक्रियाओं वाले गैल्वेनी सेल का मानक सेल-विभव परिकलित कीजिए।

(i) $2Cr\left( s \right){\text{ }} + {\text{ }}3C{d^{2 + }}\left( {aq} \right){\text{ }} \to {\text{ }}2C{r^{3 + }}\left( {aq} \right){\text{ }} + {\text{ }}3Cd$

उपर्युक्त अभिक्रियाओं के लिए Δr G- एवं साम्य स्थिरांकों की भी गणना कीजिए।

उत्तर: सेले को निम्न प्रकार प्रदर्शित किया जा सकता है 

${\text{Cr}}\left( {{\text{s}}s} \right)\left| {{\text{C}}{{\text{r}}^{3 + }}\left( {{\text{aq}}aq} \right)\parallel {\text{C}}{{\text{d}}^{2 + }}\left( {{\text{aq}}aq} \right)} \right|{\text{Cd}}\left( {{\text{s}}s} \right)$

${E_{{\text{cell}}}^ \ominus }{ = E_R^ \ominus  - E_L^ \ominus  = E_{{\text{C}}{{\text{d}}^{2 + }}/{\text{Cd}}}^ \ominus  - E_{{\text{C}}{{\text{r}}^{3 + }}/{\text{Cr}}}^ \ominus }$

${ =  - 0.40 - \left( { - 0.74} \right) =  + 0.34{\text{V}}}$

$\therefore {\text{}}\Delta_r{G^ \ominus } =  - nFE_{{\text{cell}}}^ \ominus  =  - 6 \times 96500 \times 0.34$

$\left( {F = 96500{\text{Cmo}}{{\text{l}}^{ - 1}}} \right)$

$=  - 196860{\text{CVmo}}{{\text{l}}^{ - 1}} =  - 196860{\text{Jmo}}{{\text{l}}^{ - 1}}$

$=  - 196.86{\text{kJmo}}{{\text{l}}^{ - 1}}$

$\because {\text{}}\Delta_r{G^ \ominus } =  - 2.303RT{\text{lo}}{{\text{g}}_{10}}K$

$\therefore {\text{}} - 196860 =  - 2.303 \times 8.314 \times 298 \times {\text{lo}}{{\text{g}}_{10}}K$

${\text{orlo}}{{\text{g}}_{10}}K = \dfrac{{196860}}{{2.303 \times 8.314 \times 298}} = 34.5014$

$\therefore {\text{}}K = {\text{antilo}}{{\text{g}}_{10}}\left( {34.5014} \right) = 3.172 \times {10^{34}}$

(ii) $F{e^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}Ag + {\text{ }}\left( {aq} \right){\text{ }} \to {\text{ }}F{e^{3 + }}\left( {aq} \right){\text{ }} + {\text{ }}Ag\left( s \right)$

उत्तर: सेल को निम्न प्रकार प्रदर्शित कर सकते हैं-

$F{e^{2 + }}\left( {aq} \right){\text{ }}\left| {\left| {{\text{ }}F{e^{3 + }}\left( {aq} \right){\text{ }}} \right|} \right|{\text{ }}A{g^ + }\left( {aq} \right)|{\text{ }}Ag\left( s \right)$

सेल अभिक्रिया निम्न है-

$F{e^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}A{g^ + }\left( {aq} \right){\text{ }}---{\text{ }}F{e^{3 + }}\left( {aq} \right){\text{ }} + {\text{ }}Ag\left( s \right)$

इसलिए $n = 1$

$E_{{\text{cell}}}^ \ominus$=$E_{{\text{Ag}} + /{\text{Ag}}}^ \ominus$     -    $E_{{\text{fe/Fe}}}^ \ominus$ 

$=  + 0.80{\text{ }}--{\text{ }}\left( { + 0.77} \right){\text{ }} = {\text{ }} + 0.003V$

∆r${G^ \ominus }$ $=  - nF$$E_{{\text{cell}}}^ \ominus$ ${\Delta _r}$

${ =  - 1{\text{ }} \times 96500{\text{ }} \times 0.003}$ 

${ =  - {\text{ }}2895CV{\text{ }}mo{l^{ - 1}} = {\text{ }} - 2895J{\text{ }}mo{l^{ - 1}}}$

${ =  - 2895KJ{\text{ }}mo{l^{ - 1}}}$

 ${G^ \ominus }$  $=  - {\text{ }}2.303{\text{ }}RT{\text{ }}lo{g_{10}}K$

$ - 2895 =  - 2303{\text{ }}*{\text{ }}8.314 \times 298 \times lo{g_{10}}Klo{g_{10}}K = \dfrac{{2895}}{{2.303}} \times 8.314{\text{ }} \times {\text{ }}298 = 0.5074K = antilog{\text{ }}\left( {0.5074{\text{ }}} \right){\text{ }} = {\mathbf{3}}.{\mathbf{22}}$

5. निम्नलिखित सेलों की 298K पर नेर्नुस्ट समीकरण एवं emf लिखिए।

1. $Mg\left( s \right){\text{ }}\left| {{\text{ }}M{g^{2 + }}\left( {0001{\text{ }}M} \right){\text{ }}} \right|\left| {{\text{ }}C{u^{2 + }}\left( {0.0001{\text{ }}M} \right){\text{ }}} \right|{\text{ }}Cu\left( s \right)$

उत्तर: $n = 2$सेल अभिक्रिया निम्न प्रकार है –
$Mg\left( s \right){\text{ }} + {\text{ }}C{u^{2 + }}\left( {0.0001{\text{ }}M} \right){\text{ }} \to {\text{ }}M{g^{2 + }}\left( {0.001{\text{ }}M} \right){\text{ }} + {\text{ }}Cu{\text{ }}\left( s \right)$

इसलिए n = 2,

इसके अनुसार नेर्नस्ट समीकरण निम्नवत् होगी –

${\text{Mg}}\left( s \right) + {\text{C}}{{\text{u}}^{2 + }}\left( {0.0001{\text{M}}} \right) \to {\text{M}}{{\text{g}}^{2 + }}\left( {0.001{\text{M}}} \right) + {\text{Cu}}\left( s \right)$ इसलिए ,

इसके अनुसार नेर्नस्ट समीकरण निम्नवत् होगी-

${{E_{{\text{cell}}}}}{ = E_{{\text{cell}}}^ \ominus  - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{\left[ {{\text{M}}{{\text{g}}^{2 + }}} \right]}}{{\left[ {{\text{C}}{{\text{u}}^{2 + }}} \right]}}}$

${{E_{{\text{cell}}}}}{ = \left( {E_{{\text{C}}{{\text{u}}^{2 + }}/{\text{Cu}}}^ \ominus  - {E^ \ominus }{\text{M}}{{\text{g}}^{2 + }}/{\text{Mg}}} \right) - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{0.001}}{{0.0001}}}$

${ = \left[ { + 0.34 - \left( { - 2.37} \right)} \right] - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}10}$

${ = 2.71 - 0.0295 = 2.68{\text{V}}}$

$= 0.44 - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}{10^{ - 3}}$$n = 2$

2. $Fe\left( s \right){\text{ }}\left| {{\text{ }}F{e^{2 + }}\left( {0.001{\text{ }}M} \right){\text{ }}} \right|\left| {{\text{ }}{H^ + }\left( {1{\text{ }}M} \right){\text{ }}} \right|{\text{ }}{H_2}\left( g \right){\text{ }}\left( {1{\text{ }}bar} \right){\text{ }}|{\text{ }}Pt\left( s \right)$

उत्तर: सेल अभिक्रिया निम्न है –

$Fe\left( s \right){\text{ }} + {\text{ }}2{H^ + }\left( {1M} \right) \to {\text{ }}F{e^{2 + }}\left( {0.001M} \right) + {\text{ }}{H_2}\left( {1bar} \right)$

इसलिए ,

इस सेल के epf के लिए नेर्नस्ट समीकरण निम्न होगी –

${E_{{\text{cell}}}} = \left( {E_{{{\text{H}}^ + }/\dfrac{1}{2}{{\text{H}}_2}}^ \ominus  - E_{{\text{F}}{{\text{e}}^{2 + }}/{\text{Fe}}}^ \ominus } \right) - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{\left[ {{\text{F}}{{\text{e}}^{2 + }}} \right] \times {P_{{{\text{H}}_2}}}}}{{{{\left[ {{{\text{H}}^ + }} \right]}^2}}}$

$\therefore {\text{}}{E_{{\text{cell}}}} = \left[ {0 - \left( { - 0.44} \right)} \right] - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{0.001 \times 1}}{{{{(1)}^2}}}$

$= 0.44 - \dfrac{{0.059}}{2} \times \left( { - 3} \right) = 0.529{\text{V}}$

3. $Sn\left( 8 \right){\text{ }}\left| {{\text{ }}S{n^{2 + }}\left( {0.050{\text{ }}M} \right){\text{ }}} \right|{\text{ }}{H^ + }\left( {0.020{\text{ }}M} \right){\text{ }}\left| {{\text{ }}{H_2}\left( g \right){\text{ }}\left( {1{\text{ }}bar} \right){\text{ }}} \right|{\text{ }}Pt\left( s \right)$

उत्तर: सेल अभिक्रिया निम्न है –

$Sn\left( s \right){\text{ }} + {\text{ }}2{H^ + }\left( {0.020M} \right){\text{ }} \to {\text{ }}S{n^{2 + }}\left( {0.050M} \right) + {\text{ }}{H_2}\left( {1{\text{ }}bar} \right)$

इसलिए $n = 2$,

इसके अनुसार, नेर्नस्ट समीकरण निम्न होगी –

${{E_{{\text{cell}}}}}{ = }{ - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{\left[ {{\text{S}}{{\text{n}}^{2 + }}} \right] \times p{{\text{H}}_2}}}{{{{\left[ {{{\text{H}}^ + }} \right]}^2}}}}$

${\therefore {\text{}}{E_{{\text{cell}}}}}$${ = \left[ {0 - \left( { - 0.14} \right)} \right] - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{0.050 \times 1}}{{{{(0.020)}^2}}}}$ 

$= 0.14 - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}125 = 0.078{\text{V}}$

4. $Pt\left( s \right){\text{ }}\left| {{\text{ }}B{r^ - }\left( {0.010{\text{ }}M} \right)} \right|B{r_2}\left( l \right){\text{ }}\left| {\left| {{\text{ }}{H^ + }\left( {0.030{\text{ }}M} \right){\text{ }}} \right|{\text{ }}{H_2}\left( g \right){\text{ }}\left( {1{\text{ }}bar} \right){\text{ }}} \right|{\text{ }}Pt{\text{ }}\left( s \right)$

उत्तर: सेल अभिक्रिया निम्न है –

$2B{r^ - }\left( {0.010{\text{ }}M} \right){\text{ }} + {\text{ }}2{H^ + }\left( {0.030{\text{ }}M} \right){\text{ }} \to {\text{ }}B{r_2}\left( l \right){\text{ }} + {\text{ }}{H_2}\left( {1{\text{ }}bar} \right)$

इसलिए $n = 2$,

सेल के लिए नेर्नस्ट समीकरण के अनुसार emf निम्न है –

${E_{{\text{cell}}}} = \left[ {E_{{{\text{H}}^ + }/\dfrac{1}{2}{{\text{H}}_2}}^ \ominus  - {\text{E}}_{\dfrac{1}{2}{\text{B}}{{\text{r}}_2}/{\text{B}}{{\text{r}}^ \ominus }}^ \ominus } \right] - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{{p{{\text{H}}_2}}}{{{{\left[ {{\text{B}}{{\text{r}}^ - }} \right]}^2}{{\left[ {{{\text{H}}^ + }} \right]}^2}}}$

${ = \left[ {0 - \left( { + 1.08} \right)} \right] - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\dfrac{1}{{{{(0.010)}^2}{{(0.030)}^2}}}}$ 

${ =  - 1.08 - \dfrac{{0.059}}{2}{\text{lo}}{{\text{g}}_{10}}\left( {1.11 \times {{10}^7}} \right)}$

${ =  - 1.08 - 0.208 =  - 1.288{\text{V}}}$

5. घड़ियों एवं अन्य युक्तियों में अत्यधिक उपयोग में आने वाली बटन सेलों में निम्नलिखित अभिक्रिया होती है –

उत्तर:

$Zn\left( s \right){\text{ }} + {\text{ }}A{g_2}O\left( s \right){\text{ }} + {\text{ }}{H_2}O\left( l \right){\text{ }} \to {\text{ }}Z{n^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}2Ag\left( s \right){\text{ }} + {\text{ }}2O{H^ - }\left( {aq} \right)$

6. अभिक्रिया के लिए ΔrG- एवं E– ज्ञात कीजिए।

उत्तर:

$E_{{\text{cell}}}^ \ominus  = E_{{\text{A}}{{\text{g}}_2}{\text{O}}/{\text{Ag}}}^ \ominus  - E_{{\text{Z}}{{\text{n}}^{2 + }}/{\text{Zn}}}^ \ominus  =  + 0.344 - \left( {0.76} \right) = 1.104V$
सेल अभिक्रिया के लिए, $n=2$

$\therefore \Delta_{r} G-=-n F E^{-} \text {cell }$

$\therefore \Delta_{r} G-=-2 \times 96500 \times 1.104$

$=-2.13 \times 10^{5} \mathrm{CV} \mathrm{mol}^{-1}$

$=-2.13 \times 10 \mathrm{~J} \mathrm{~mol}^{-1}$

7. किसी विद्युत-अपघट्य के विलयन की चालकता एवं मोलर चालकता की परिभाषा दीजिए। सान्द्रता के साथ इनके परिवर्तन की विवेचना कीजिए।

उत्तर: विद्युत-अपघट्य के विलयन की चालकता (Conductivity of the solution of an electrolyte) – यह प्रतिरोध R का व्युत्क्रम होती है और इसे उस सरल रूप में परिभाषित किया जा सकता है जिससे धारा किसी चालक में प्रवाहित होती है।

$c{\text{ }} =$$\dfrac{1}{{\text{R}}}$=$\dfrac{{\text{A}}}{{{\text{pl}}}}$

$k{\text{ }} =$$\dfrac{{\text{A}}}{{\text{l}}}$

यहाँ $k$ विशिष्ट चालकता है। चालकता का $SI$ मात्रक सीमेन्ज (Siemens) है जिसे प्रतीक  से निरूपित किया जाता है और यह ${\text{oh}}{{\text{m}}^{{\text{ - 1}}}}$ या ${\Omega ^{ -

1}}$ के तुल्य होता है।

मोलर चालकता (Molar conductivity) – वह चालकता जो $1$ मोल विद्युत-अपघट्य को विलयन में घोलने पर समस्त आयनों द्वारा दर्शायी जाती है,और वह मोलर चालकता कहलाती है, इसे ${\Delta _m}$ (लैम्ब्डा) से भी व्यक्त किया जाता है। यदि विद्युत-अपघट्य विलयन के $V{\text{ }}c{m^3}$ में विद्युत-अपघट्य के $1{\text{ mol}}$ उपस्थित हों, तब

${\Delta _m} = {\text{ }}K{\text{ }} \times V$

${ = \dfrac{{k \times 1000}}{{{\text{Conductivity}}}} = \dfrac{{k \times 1000}}{M}}$

इसकी इकाई ${\text{oh}}{{\text{m}}^{{\text{ -

1}}}}{\text{c}}{{\text{m}}^{\text{2}}}{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}$ या ${\text{S

c}}{{\text{m}}^{\text{2}}}{\text{mo}}{{\text{l}}^{{\text{ - 1}}}}$ है।

सान्द्रता के साथ चालकता तथा मोलर चालकता में परिवर्तन

(Variation of Conductivity and Molar Conductivity with Concentration)

विद्युत-अपघट्य की सान्द्रता में परिवर्तन के साथ-साथ चालकता एवं मोलर चालकता दोनों में परिवर्तन होता है। दुर्बल एवं प्रबल दोनों प्रकार के विद्युत-अपघट्यों की सान्द्रता घटाने पर चालकता सदैव घटती है। इसकी इस तथ्य से व्याख्या की जा सकती है कि तनुकरण (dilution) करने पर प्रति इकाई आयतन में विद्युत धारा ले जाने वाले आयनों की संख्या घट जाती है। किसी भी सान्द्रता पर विलयन की चालकता उस विलयन के इकाई आयतन का चालकत्व होता है जिसे परस्पर इकाई दूरी पर स्थित एवं इकाई अनुप्रस्थ काट क्षेत्रफल वाले दो प्लैटिनम इलेक्ट्रोडों के मध्य रखा गया हो।

यह निम्नलिखित समीकरण से स्पष्ट है –

$C =$$\dfrac{{kl}}{l}$

($A$ एवं $l$ दोनों ही उपयुक्त इकाइयों $m$ या $cm$ में हैं)

किसी दी गई सान्द्रता पर एक विलयन की मोलर चालकता उस विलयन के $V$ आयतन का चालकत्व है जिसमें विद्युत-अपघट्य का एक मोल घुला हो तथा जो एक-दूसरे से इकाई दूरी पर स्थित हो, $A$ अनुप्रस्थ काट क्षेत्रफल वाले दो इलेक्ट्रोडों के मध्य रखा गया हो। अतः

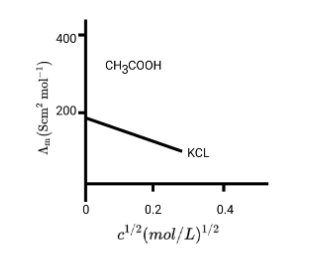
जलीय विलयन में पोटैशियम क्लोराइड्ड (प्रबल विद्युत-अपघट्य) के लिए मोलर चालकता के विपरीत $c^{1 / 2}$ का आलेख।
${\Delta _m} = \dfrac{{kA}}{l} = k$

चूंकि $l{\text{ }} = {\text{ }}1$ एवं $A{\text{ }} = {\text{ }}V$ (आयतन, जिसमें विद्युत अपघट्य का एक मोल घुला है।)

${\Delta _m} = {\text{ }}k{\text{ }}V$

सान्द्रता घटने के साथ मीलर चालकता बढ़ती है। ऐसा इसलिए होता है क्योंकि वह कुल आयतन ($V$) भी बढ़ जाता है जिसमें एक मोल विद्युत अपघट्य उपस्थित होता है। यह पाया गया है कि विलयन के तनुकरण पर आयतन में वृद्धि $K$ में होने वाली कमी की तुलना में कहीं अधिक होती है।

प्रबल विद्युत-अपघट्य (Strong Electrolytes)

प्रबल विद्युत अपघट्यों के लिए Δm का मान तनुता के साथ धीरे-धीरे बढ़ता है एवं इसे निम्नलिखित समीकरण द्वारा निरूपित किया जा सकता है –

यह देखा जा सकता है कि यदि ${\Delta _m}$ को ${c^{\dfrac{1}{2}}}$ के विपरीत आरेखित किया जाए (चित्र-3) तो हमें एक सीधी रेखा प्राप्त होती है जिसका अन्त:खण्ड $A$ एवं ढाल $A$ के बराबर है। दिए गए विलायक एवं ताप पर स्थिरांक ‘A का मान विद्युत-अपघट्य के प्रकार अर्थात् विलयन में विद्युत-अपघट्य के वियोजन से उत्पन्न धनायन एवं ऋणायन के आवेशों पर निर्भर करता है। अत: $NaCl,{\text{ }}CaC{l_2},{\text{ }}MgS{O_4}$ क्रमशः $1 - 1,{\text{ }}2 - 1$ एवं $2 - 2$ विद्युत-अपघट्य के रूप में जाने जाते हैं। एक प्रकार के सभी विद्युत-अपघट्यों के लिए $A$ का मान समान होता है।

8. $298{\text{ }}K$ पर $0.20{\text{ }}M{\text{ }}KCl$ विलयन की चालकता $0.0248{\text{ Sc}}{{\text{m}}^{{\text{ - 1}}}}$ है। इसकी मोलर चालकता का परिकलन कीजिए।

उत्तर:

${{\Delta _m} = \dfrac{{k \times 1000}}{{{\text{conductivity}}}}}$ 

${ = \dfrac{{0.0248{\text{Sc}}{{\text{m}}^{ - 1}} \times 1000{\text{c}}{{\text{m}}^3}{\text{}}{{\text{L}}^{ - 1}}}}{{0.20{\text{mol}}{{\text{L}}^{ - 1}}}} = 124{\text{Sc}}{{\text{m}}^2}{\text{mo}}{{\text{l}}^{ - 1}}}$ 

9. $298{\text{ }}K$  पर एक चालकता सेल जिसमें $0.001{\text{ }}M{\text{ }}KCl$ विलयन है, का प्रतिरोध $1500{\text{ }}\Omega $ है। यदि $0.001{\text{ }}M{\text{ }}KCl$ विलयन की चालकता $298{\text{ }}K$ पर $0.146{\text{ }} \times {10^{ - 3}}S{\text{ }}c{m^{ - 1}}$ हो तो सेल स्थिरांक क्या है?

उत्तर:

$k{\text{ }} = \dfrac{{\mathbf{1}}}{R}x$ सेल नियतांक

∴ सेल नियतांक $= {\text{ }}K{\text{ }}R = {\text{ }}0.146 \times {10^{ - 3}} \times

1500{\text{ }} = {\text{ }}0.219{\text{ }}c{m^{ - 1}}$

10. $298{\text{ }}K$  पर सोडियम क्लोराइड की विभिन्न सान्द्रताओं पर चालकता का मापन किया गया जिसके आँकड़े अग्रलिखित हैं –

${{\text{M}}}:{0.001}{0.010}{0.020}{0.050}{0.100}$ 

${{{10}^2} \times k/s{\text{}}{m^{ - 1}}}:{1.237}{11.85}{23.15}{55.53}{106.74}$ 

सभी सान्द्रताओं के लिए ${{\mathbf{\Lambda }}_m}$ का परिकलन कीजिए एवं ${{{\Lambda }}_m}$ तथा $c\dfrac{L}{2}$ के मध्य एक आलेख खींचिए। ${{\Lambda }}_m^ \circ$ का मान ज्ञात कीजिए।

उत्तर:

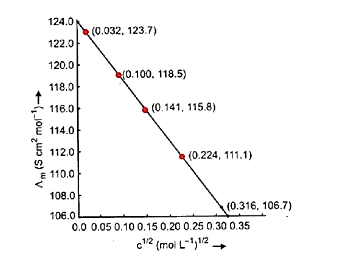
सीधी रेखा को पीछे तक खींचने पर यह ${\Delta _m}$ अक्ष पर $124.0{\text{ }}S{\text{ }}c{m^2}mo{l^{ - 1}}$ पर मिलती है। यह  का मान है।

11. $0.00241{\text{ }}M$ ऐसीटिक अम्ल की चालकता $7.896 \times {10^{ - 5}}S{\text{ }}c{m^{ - 1}}$ है। इसकी मोलर चालकता को परिकलित कीजिए। यदि ऐसीटिक अम्ल के लिए   का मान $390.5{\text{ }}S{\text{ }}c{m^2}mo{l^{ - 1}}$ हो तो इसका वियोजन स्थिरांक क्या है?

उत्तर:
${ \wedge _{\text{m}}^c = \dfrac{{k \times 1000}}{{{\text{conductivity}}}}}{ = \dfrac{{7.896 \times {{10}^{ - 5}} \times 1000}}{{0.00241}} = 32.763{\text{Sc}}{{\text{m}}^2}{\text{mo}}{{\text{l}}^{ - 1}}}$ 

${a = \dfrac{{ \wedge _m^ \circ }}{{ \wedge _m^ \circ }} = \dfrac{{32.763}}{{390.5}} = 0.084}$ 

${K = \dfrac{{{a^2}c}}{{\left( {1 - a} \right)}} = \dfrac{{{{(0.084)}^2} \times 0.00241}}{{1 - 0.084}}}$ 

  ${ = \dfrac{{{{(0.084)}^2} \times 0.00241}}{{0.916}} = 1.86 \times {{10}^{ - 5}}}$ 

12. निम्नलिखित के अपचयन के लिए कितने आवेश की आवश्यकता होगी?

(i) $1$ मोल $A{l^{3 + }}$ को $Al$ में

उत्तर: ${\text{Al}}_{1{\text{mol}}}^{3 + } + \mathop {{{\text{e}}^ - }}\limits_{3{\text{mol}}}  \to {\text{Al}}$

इसलिए आवश्यक आवेश $= 3{\text{F}}$, क्योंकि एक मोल इलेक्ट्रॉन का आवेश $1{\text{F}}$ होता है।

(ii) $1$ मोल $C{u^{2 + }}$ को $Cu$ में

उत्तर: $\mathop {{\text{C}}{{\text{u}}^{2 + }}}\limits_{1{\text{mol}}}  + \mathop {2{e^ - }}\limits_{2{\text{mol}}}  \to {\text{Cu}}$

आवश्यक आवेश $= 2F$

(iii) $1$ मोल $Mn{O^ - }_4$ को $M{n^{2 + }}$ में
उत्तर:

${{\text{MnO}}_4^ -  + 8{{\text{H}}^ + } + 5{n^ - }}$

${1{\text{mol}}}{5{\text{mol}}}$ 

${\text{M}}{{\text{n}}^{2 + }} + 4{{\text{H}}_2}{\text{O}}$

आवश्यक आवेश $= 5F$

13. निम्नलिखित को प्राप्त करने में कितने फैराडे विद्युत की आवश्यकता होगी?

(i) गलित $CaC{l_2}$ से $20.0{\text{ }}g{\text{ }}Ca$

उत्तर:

${\text{CaC}}{{\text{l}}_2} \to {\text{Ca}}$

${\text{C}}{{\text{a}}^{2 + }} + 2{e^ - } \to {\text{Ca}}$

या $1$$mole{\text{ }}2{\text{ }}moles{\text{ }}1{\text{ }}mole$

या $40{\text{ }}g{\text{ }}2{\text{ }}moles$

या $20{\text{ }}g{\text{ }}1{\text{ }}mole$$20{\text{g}}$

$\therefore$ आवश्यक विद्युत की मात्रा $= 1{\text{F}}$

(ii) गलित $A{l_2}{O_3}$ से $40.0{\text{ }}g{\text{ }}Al$

उत्तर: $\dfrac{1}{2}{\text{A}}{{\text{l}}_2}{{\text{O}}_3} \to {\text{Al}}$

या ${\text{A}}{{\text{l}}^{3 + }} + 3{e^ - } \to {\text{Al}}$

या $1{\text{ }}mole{\text{ }}3{\text{ }}moles{\text{ }}1{\text{ }}mole$

या $26.98{\text{g}}3$ moles $26.98{\text{g}}$

$\because {\text{Al}}$ के $26.98$ ग्राम के लिए आवश्यक विद्युत $= 3{\text{F}}$

$\therefore {\text{Al}}$ के $40$ ग्राम के लिए आवश्यक विद्युत $= \dfrac{3}{{26.98}} \times 40 = 4.448{\text{F}}$

14. निम्नलिखित को ऑक्सीकृत करने के लिए कितने कूलॉम विद्युत आवश्यक है?

1. $1$ मोल ${H_2}O$ को ${O_2}$ में।

उत्तर: $1{\text{ }}mol{\text{ }}{H_2}O$ के लिए इलेक्ट्रोड अभिक्रिया इस प्रकार दी जाती है –
${H_2}O{\text{ }} \to {\text{ }}{H_2} + \dfrac{1}{2}{O_2}$

अर्थात् ${O^{2 - }} \to \dfrac{1}{2}{O_2} + {\text{ }}2{e^ - }$

∴ आवश्यक विद्युत की मात्रा $= 2F = 2 \times 96500C = 193000C$

2. $1$ मोल $FeO$ को $F{e_2}{O_3}$ में। 

उत्तर: $1{\text{ }}mol{\text{ }}FeO$के लिए इलेक्ट्रोड अभिक्रिया इस प्रकार दी जाती है –
$FeO{\text{ }} \to \dfrac{1}{2}F{e_2}{O_3}$

अर्थात् $F{e^{2 + }} \to {\text{ }}F{e^{3 + }} + {\text{ }}{e^ - }$

∴ आवश्यक विद्युत की मात्रा $= 1F = 96500C$

15. $Ni{\left( {N{O_3}} \right)_2}$ के एक विलयन का प्लैटिनम इलेक्ट्रोडों के बीच $5$ ऐम्पियर की धारा प्रवाहित करते हुए $20$ मिनट तक विद्युत-अपघटन किया गया। $Ni$ की कितनी मात्रा कैथोड पर निक्षेपित होगी?

उत्तर:

अभिक्रिया निम्न प्रकार सम्पन्न होती है –

$N{i^{2 + }} + {\text{ }}2{e^{--}} \to {\text{ }}Ni$

$Ni$ का परमाणु भार $= 58.70$

${\text{Ni}}$ का तुल्यांकी भार $\frac{परमाण भार}{संयोजी इलेक्ट्रानों की संख्या}$=$\dfrac{{58.70}}{2}=29.35$

विद्युत अपघटन के फैराडे के प्रथम नियमानुसार,

$W{ = Z.I.t = \dfrac{{weighedweight{\text{}}}}{{96500}} \times I \times t}$ 

${ = \dfrac{{29.35}}{{96,500}} \times 5 \times 20 \times 60 = 1.825{\text{g}}}$ 

16. $ZnS{O_4},{\text{ }}AgN{O_3}$ एवं $CuS{O_4}$ विलयन वाले तीन विद्युत-अपघटनी सेलों $A,{\text{ }}B,{\text{ }}C$ को श्रेणीबद्ध किया गया एवं $1.5$ ऐम्पियर की विद्युत धारा, सेल B के कैथोड पर $145$ सिल्वर निक्षेपित होने तक लगातार प्रवाहित की गई। विद्युत धारो कितने समय तक प्रवाहित हुई? निक्षेपित कॉपर एवं जिंक को द्रव्यमान क्या होगा ?

उत्तर: 

${\text{A}}{{\text{g}}^ + } + {e^ - } \to {\text{Ag}}$

$108{\text{gAg}}$ निक्षेपित होता है $= 1{\text{F}} = 96500{\text{C}}$

$\therefore {\text{}}1.45{\text{gAg}}$ निक्षेपित होगा $= \dfrac{{96500}}{{108}} \times 1.45 = 1295.6{\text{C}}$

$Q = It$

${t = \dfrac{Q}{I} = \dfrac{{1295.6}}{{1.5}} = 863.7{\text{s}} = 14{\text{min}}24s}$ 

${{\text{C}}{{\text{u}}^{2 + }} + 2{e^ - } \to {\text{Cu}}}$ 

अर्थात् $2 \times 96500{\text{C}},{\text{Cu}}$ निक्षेपित करता है $= 63.5{\text{g}}$
अत: $1295.6{\text{C}},{\text{Cu}}$ निक्षेपित करेगा $= \dfrac{{63.5 \times 1295.6}}{{2 \times 96500}} = 0.4263{\text{g}}$

इसी प्रकार, ${\text{Z}}{{\text{n}}^{2 + }} + 2{e^ - } \to {\text{Zn}}$

निक्षेपित जिंक का द्रव्यमान $= \dfrac{{65.4 \times 1295.6}}{{2 \times 96500}} = 0.44{\text{g}}$

17. तालिका $3.1$ (पाठ्यपुस्तक) में दिए गए मानक इलेक्ट्रोड विभवों की सहायता से अनुमान लगाइए कि क्या निम्नलिखित अभिकर्मकों के बीच अभिक्रिया सम्भव है?

(i) $F{e^{3 + }}\left( {aq} \right)$ और ${I^ - }\left( {aq} \right)$

उत्तर:  कोई अभिक्रिया तब सम्भव होती है, जब $E_{{\text{cell}}}^ \ominus$ का मान धनात्मक होता है।

(i) प्रश्नानुसार, अभिक्रिया निम्न है-

${\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right) + {{\text{I}}^ - }\left( {aq} \right) \to {\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right) + {{\text{I}}_2}\left( s \right)$

इसके अनुसार सेल निम्न होगा-

${{\text{Pt}},{{\text{I}}_2}\left( s \right)\left| {{{\text{I}}^ - }\left( {aq} \right)\parallel {\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right)} \right|{\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right)}$ 

${\therefore {\text{}}}{E_{{\text{cell}}}^ \ominus  = {\text{E}} - E_{\dfrac{1}{2}{{\text{I}}_2}/{{\text{I}}^ - }}^ \circ  = 0.77 - \left( { + 0.54} \right) =  + 0.23{\text{V}}}$

चूँकि $E_{{\text{cell}}}^ \ominus$ का मान धनात्मक है, अतः अभिक्रिया सम्भव है।

(ii) $A{g^ + }\left( {aq} \right)$ और $Cu{\text{ }}\left( s \right)$

उत्तर:  अभिक्रिया निम्न है-

${\text{ - Cu}}\left( s \right) + {\text{A}}{{\text{g}}^ + }\left( {aq} \right) \to {\text{C}}{{\text{u}}^ + }\left( {aq} \right) + {\text{Ag}}\left( s \right)$

अभिक्रिया सेल निम्न है-

${{\text{Cu}}\left( s \right)\left| {{\text{C}}{{\text{u}}^{2 + }}\left( {aq} \right)\parallel {\text{A}}{{\text{g}}^ + }\left( {aq} \right)} \right|{\text{Ag}}\left( s \right)}$ 

${\therefore E_{{\text{cell}}}^ \ominus  = {\text{E}}_{{\text{A}}{{\text{g}}^ + }/{\text{Ag}}}^ \circ  - E_{{\text{C}}{{\text{u}}^{2 + }}/{\text{Cu}}}^ \circ  = 0.80 - \left( { + 0.34} \right) =  + 0.46{\text{V}}}$

चूँकि $E_{{\text{cell}}}^ \ominus$ धनात्मक है, अतः अभिक्रिया होगी।

(iii) $F{e^{3 + }}\left( {aq} \right)$ और $B{r^ - }\left( {aq} \right)$

उत्तर:  अभिक्रिया निम्न है-

${\text{B}}{{\text{r}}^ - }\left( {aq} \right) + {\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right) \to \dfrac{1}{2}{\text{B}}{{\text{r}}_2}\left( {aq} \right) + {\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right)$

अत: सेल निम्न होगा-

${{\text{B}}_{\text{i}}^ - \left( {aq} \right)\left| {\dfrac{1}{2}{\text{B}}{{\text{r}}_2}\left( {aq} \right)\parallel {\text{F}}{{\text{e}}^{3 - }}\left( {aq} \right)} \right|{\text{F}}{{\text{e}}^{2 - }}\left( {aq} \right)}$ 

${E_{{\text{cell}}}^ \ominus  = E_{{\text{F}}{{\text{e}}^{3 - }}/{\text{F}}{{\text{e}}^{2 - }}}^ \circ  - E_{\dfrac{1}{2}{\text{BrB}}{{\text{r}}^ - }}^ \circ  = 0.77 - \left( { + 1.09} \right) =  - 0.32V}$ 

चूँकि $E_{{\text{cell}}}^ \ominus$ ॠणात्मक है, अत: अभिक्रिया सम्भव नहीं है।

(iv) $Ag{\text{ }}\left( s \right)$ और $F{e^{3 + }}\left( {aq} \right)$

उत्तर:  अभिक्रिया निम्न है--

${\text{Ag}}\left( s \right) + {\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right) \to {\text{A}}{{\text{g}}^ + }\left( {aq} \right) + {\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right)$

सम्बन्धित सेल निम्न होगा-

${{\text{Ag}}\left( a \right)\left| {{\text{A}}{{\text{g}}^ + }\left( {aq} \right)\parallel {\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right)} \right|{\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right)}$ 

${E_{{\text{cell}}}^ \ominus  = {\text{E}} - E_{{\text{A}}{{\text{g}}^ + }/{\text{Ag}}}^ \circ  = 0.77 - \left( { + 0.80} \right) =  - 0.03{\text{V}}}$ 

चूँकि $E_{{\text{cell}}}^ \ominus$ का मान ऋणात्मक है, अत: अभिक्रिया नहीं होगी।

(v) $B{r_2}\left( {aq} \right)$ और $F{e^{2 + }}\left( {aq} \right)$

उत्तर:  अभिक्रिया निम्न है-

${\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right) + \dfrac{1}{2}{\text{B}}{{\text{r}}_2}\left( {aq} \right) \to {\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right) + {\text{B}}{{\text{r}}^ - }\left( {aq} \right)$

सम्बन्धित सेल निम्न है-

${{\text{F}}{{\text{e}}^{2 + }}\left( {aq} \right)\left| {{\text{F}}{{\text{e}}^{3 + }}\left( {aq} \right)!} \right|\dfrac{1}{2}{\text{B}}{{\text{r}}_2}\left( {aq} \right)\mid {\text{B}}{{\text{r}}^ - }\left( {aq} \right)}$ 

${E_{{\text{cell}}}^ \ominus  = {\text{E}} - E_{{\text{F}}{{\text{e}}^{3 + }}/{\text{F}}{{\text{e}}^{2 + }}}^ \circ  = 1.09 - \left( { + 0.77} \right) =  + {\text{}}0.31V}$

चूँकि $E_{{\text{cell}}}^ \ominus$ का मान धनात्मक है, अतः अभिक्रिया होगी।

18. निम्नलिखित में से प्रत्येक के लिए विद्युत-अपघटन से प्राप्त उत्पाद बताइए –

 1. सिल्वर इलेक्ट्रोडों के साथ $AgN{O_3}$ का जलीय विलयन

उत्तर: 

$AgN{O_3}\left( {aq} \right){\text{ }} \to {\text{ }}A{g^ + }\left( {aq} \right){\text{ }} + {\text{ }}N{O^{ - 3}}\left( {aq} \right)$

${H_2}O \to {H^ + } + {\text{ }}OH$ 

कैथोड पर : क्युकि सिल्वर का अपचयन विभव $\left( { + 0.80{\text{ }}V} \right)$ जल $\left( { - 0.830{\text{ }}V} \right)$ से अधिक है, इसलिए $A{g^ + }$ वरीयता के आधार पर अपचयित होगा और सिल्वर धातु कैथोड पर जमा होगा।

$A{g^ + }\left( {aq} \right){\text{ }} + {\text{ }}{e^{--{\text{ }}}} \to {\text{ }}Ag{\text{ }}\left( s \right)$

ऐनोड पर : निम्न अभिक्रिया होगी –

${H_2}O{\text{ }}\left( l \right){\text{ }} \to {\text{ }}\dfrac{1}{2}{O_2}\left( g \right){\text{ }} + {\text{ }}2{H^{ + {\text{ }}}}\left( {aq} \right)$

$N{O^ - }_3\left( {aq} \right){\text{ }} \to {\text{ }}N{O_3} + {\text{ }}{e^--}$

$Ag\left( s \right){\text{ }} \to {\text{ }}A{g^ + }{\text{ }}\left( {aq} \right){\text{ }} + {\text{ }}{e^--}$

इन अभिक्रियाओं में कॉपर का अपचयन विभव न्यूनतम है। इसलिए सिल्वर स्वयं ऐनोड पर ऑक्सीकरण के फलस्वरूप  में परिवर्तित हो जायेगी और  आयन विलयन में चले जायेंगे।
$Ag\left( s \right){\text{ }} \to {\text{ }}A{g^ + }\left( {aq} \right){\text{ }} + {\text{ }}{e^ - }$

2. प्लैटिनम इलेक्ट्रोडों के साथ $AgN{O_3}$ का जलीय विलयन
उत्तर: 

कैथोड पर : सिल्वर आयने अपचयित होंगे और सिल्वर धातु जमा होगी।

ऐनोड पर : क्युकि जल का अपचयन विभव $N{O^ - }_3$ आयनों से कम होता है, इसलिए जल वरीयता के आधार पर ऑक्सीकृत होगा तथा ऑक्सीजन मुक्त होगी।

${H_2}O{\text{ }}\left( l \right){\text{ }} \to {\text{ }}\dfrac{1}{2}{O_2}\left( g \right){\text{ }} + {\text{ }}2{H^ + }\left( {aq} \right){\text{ }} + {\text{ }}2{e^ - }$

3. प्लैटिनम इलेक्ट्रोडों के साथ ${H_2}S{O_3}$ का तनु विलयन

उत्तर: प्लैटिनम इलेक्ट्रोडों के साथ ${H_2}S{O_4}$ के तनु विलयन का विद्युत-अपघटन

${H_2}S{O_4}\left( {aq} \right){\text{ }} \to {\text{ }}2{H^ + }\left( {aq} \right){\text{ }} + {\text{ }}S{O^{2 - }}_4\left( {aq} \right)$

${H_2}O{H^ + } + {\text{ }}O{H^ - }$

कैथोड पर : ${H^ + } + {\text{ }}{e^ - } \to {\text{ }}H$
$H{\text{ }} \to {\text{ }}{H_2}\left( g \right)$

ऐनोड पर : $O{H^ - } \to {\text{ }}OH{\text{ }} + {\text{ }}{e^ - }$

$4OH{\text{ }} \to {\text{ }}2{H_2}O{\text{ }}\left( l \right){\text{ }} + {\text{ }}{O_2}\left( g \right)$

अत: कैथोड पर $H$, तथा ऐनोड पर $O$ मुक्त होगी।

4. प्लैटिनम इलेक्ट्रोडों के साथ $CuC{l_2}$ का जलीय विलयन।

उत्तर: $CuC{l_2}\left( {aq} \right){\text{ }} \to {\text{ }}C{u^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}2C{l^ - }\left( {aq} \right)$

$2{H_2}O{H^ + } + {\text{ }}OH$

कैथोड पर : क्युकि $C{u^{2 + }}\left( { + 0.341{\text{ }}V} \right)$ का अपचयन विभव जल $\left( { - 0.83{\text{ }}V} \right)$ से अधिक होता है, इसलिए $C{u^{2 + }}$ वरीयता के आधार पर अपचयित होंगे तथा कैथोड पर कॉपर धातु जमा होगी।
$2C{u^{2 + }}\left( {aq} \right){\text{ }} + {\text{ }}2{e^ - } \to {\text{ }}Cu{\text{ }}\left( s \right)$

ऐनोड पर : निम्न अभिक्रियाओं के होने की सम्भावना है –

${H_2}O{\text{ }}\left( l \right){\text{ }} \to {\text{ }}\dfrac{1}{2}{O_2}{\text{ }}\left( g \right){\text{ }} + {\text{ }}2{H^ + }\left( {aq} \right){\text{ }} + {\text{ }}2{e^ - };$

$E^\circ {\text{ }} = {\text{ }} + 1.23{\text{ }}V$

$2Cl--{\text{ }}\left( {aq} \right){\text{ }} \to {\text{ }}C{l_2}\left( g \right){\text{ }} + {\text{ }}2{e^ - };{\text{ }}E^\circ {\text{ }} = {\text{ }} + {\text{ }}1.36V$क्युकि जल का अपचयन विभव $C{l^ - }$(जलीय) आयनों से कम होता है, इसलिए जल वरीयता के आधार पर ऐनोड पर ऑक्सीकृत होगा तथा ${O_2}$, गैस मुक्त होगी

NCERT Solutions for Class 12 Chemistry Chapter 3 Electrochemistry Hindi Medium

Chapter-wise NCERT Solutions are provided everywhere on the internet with an aim to help the students to gain a comprehensive understanding. Class 12 Chemistry Chapter 3 solution Hindi mediums are created by our in-house experts keeping the understanding ability of all types of candidates in mind. NCERT textbooks and solutions are built to give a strong foundation to every concept. These NCERT Solutions for Class 12 Chemistry Chapter 3 in Hindi ensure a smooth understanding of all the concepts including the advanced concepts covered in the textbook.

NCERT Solutions for Class 12 Chemistry Chapter 3 in Hindi medium PDF download are easily available on our official website (vedantu.com). Upon visiting the website, you have to register on the website with your phone number and email address. Then you will be able to download all the study materials of your preference in a click. You can also download the Class 12 Chemistry Electrochemistry solution Hindi medium from Vedantu app as well by following the similar procedures, but you have to download the app from Google play store before doing that.

NCERT Solutions in Hindi medium have been created keeping those students in mind who are studying in a Hindi medium school. These NCERT Solutions for Class 12 Chemistry Electrochemistry in Hindi medium pdf download have innumerable benefits as these are created in simple and easy-to-understand language. The best feature of these solutions is a free download option. Students of Class 12 can download these solutions at any time as per their convenience for self-study purposes.

These solutions are nothing but a compilation of all the answers to the questions of the textbook exercises. The answers/solutions are given in a stepwise format and very well researched by the subject matter experts who have relevant experience in this field. Relevant diagrams, graphs, illustrations are provided along with the answers wherever required. In nutshell, NCERT Solutions for Class 12 Chemistry in Hindi come really handy in exam preparation and quick revision as well prior to the final examinations.