Exam-Focused Revision Notes for CBSE Class 10 Maths Chapter 2 - Polynomials






Polynomials Class 10 Notes CBSE Maths Chapter 2 (Free PDF Download)



FAQs on Polynomials Class 10 Notes CBSE Maths Chapter 2 (Free PDF Download)
1. Find the value of “p” from the polynomial x2 + 3x + p, if one of the zeroes of the polynomial is 2.
It is given that, 2 is the zero of the polynomial.
We know that if α is a zero of the polynomial p(x), then p(α) = 0
So,Substituting x = 2 in x2 + 3x + p,
We get, 22 + 3(2) + p = 0
4 + 6 + p = 0
10 + p = 0
p = -10.
Hence the value of p is -10.
2. How many zeros do the polynomial (x – 3)2 – 4 can have? Also, find them.
Given the polynomial equation is (x – 3)2 – 4
Now, we need to expand this equation.
x2 + 9 – 6x – 4
x2– 6x + 5
As the equation has a degree of 2, it is called a quadratic polynomial. So, the number of zeroes will be 2.
Now, solve x2 - 6x + 5 = 0 to get the factors by using the factorization method.
So, x2– x – 5x + 5 = 0
x(x – 1) -5(x – 1) = 0
(x – 1)(x – 5) = 0
x = 1, x = 5
So, the factors are 1 and 5.
These are the two zeros that we need to find.
Hence it is solved.
3. What is polynomial in the context of Chapter 2 Class 10?
The term Polynomial comes from the word 'poly' which means 'many' and 'nominal' which means term. Therefore, polynomials refer to many terms. A polynomial is made up of many terms which can only be substrated, added, or multiplied. A polynomial with the highest exponent of variables is known as the degree of the polynomial. The polynomial with degree one is known as a linear, degree two is known as a quadratic, and degree three is known as a cubic polynomial.
4. What are important polynomial notes for chapter 2 class 10?
While studying Chapter 2 Class 10 Maths, it is important for you to understand the concept of the polynomial. This is an important chapter from your board examination point of view. The important polynomial notes include types of polynomials, the degree of polynomials, zeros of a polynomial, formulas, and all the algorithms. These notes will help you during your revision time as well, to help you score well during your board exam.
5. Where can I find NCERT Solutions for Class 10 Maths Chapter 2?
Vedantu provides students with the best NCERT Solutions for the Class 10 Maths Chapter 2. It provides all important questions and solutions for Chapter 2 “Polynomials''. It covers all types of questions from the board examination point of view. They have a variety of questions to help students understand the chapters and important concepts better. These solutions and questions are extremely important for all CBSE students from the viewpoint of examinations. Also, the solutions PDF is available for free download on the Vedantu mobile app.
6. How can you divide one polynomial with another polynomial?
A polynomial refers to an expression with more than two algebraic terms. It refers to the sum of many terms that have different powers of the same variable. You can divide one polynomial with another polynomial using two methods namely long division, and synthetic division. The easiest way to divide one polynomial with another polynomial is using long division. Using long division will help to test whether or not a single polynomial has another one as a factor.
7. How to ace Class 10 Maths Chapter 2?
Class 10 Maths Chapter 2 is an important topic from the examination point of view. Students can rely on the study materials available on the official website of Vedantu or their app and they are available free of cost. One of the most important ways to ace Chapter 2 is to complete all the NCERT questions and answers and carry out thorough revision. Revision is an important part of exam preparation. By completing solutions and exercises the students can ace their Class 10 Maths Chapter 2.




















 Watch Video
Watch Video


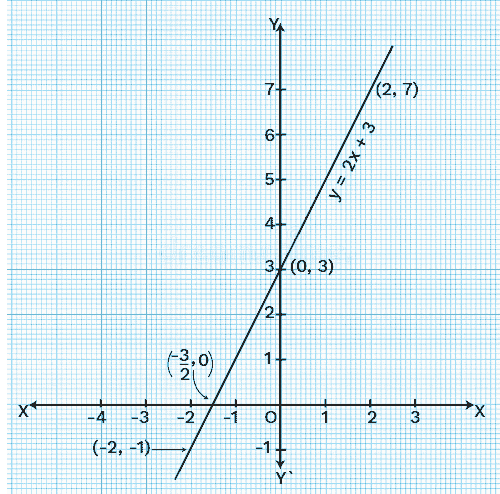
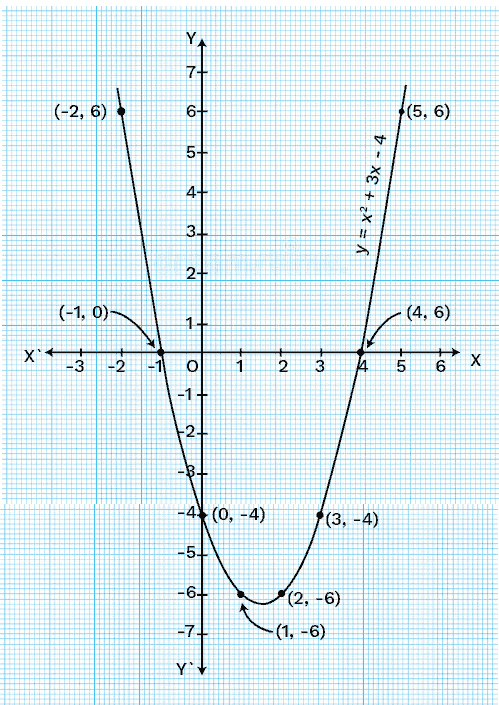
![The graph cuts \[\text{x-}\]axis at two distinct points \[\text{A}\]and \[\text{A }\!\!'\!\!\text{ }\]](https://www.vedantu.com/seo/content-images/0380daf6-a4f0-4033-8282-1de34df19e30.png)
![The graph cuts \[\text{x-}\]axis at two distinct points \[\text{A}\]and \[\text{A }\!\!'\!\!\text{ }\]](https://www.vedantu.com/seo/content-images/6376383b-5d4f-4b4b-80a4-0647b3ce8afa.png)
![The graph cuts the \[\text{x-}\]axis at exactly one point](https://www.vedantu.com/seo/content-images/a67baba1-1ff5-4702-bd38-ce6449198a5e.png)
![The graph cuts the \[\text{x-}\]axis at exactly one point](https://www.vedantu.com/seo/content-images/b06427b5-04aa-4d76-a7f6-5864941d830d.png)
![the graph is either completely above the \[\text{x-}\]axis or completely below the \[\text{x-}\]axis](https://www.vedantu.com/seo/content-images/d09d2a49-7d52-4f90-a995-fa4774936bad.png)
![the graph is either completely above the \[\text{x-}\]axis or completely below the \[\text{x-}\]axis](https://www.vedantu.com/seo/content-images/c813bd14-5408-4d20-bef4-1b6047c29661.png)












