Question

A block slides down the frictionless ramp shown in figure. Use the law of conservation of energy to find its speed when it gets to the bottom. $\left(g=10 \mathrm{m} \mathrm{s}^{-2}\right)$,

(A) $20 \mathrm{m} / \mathrm{s}$
(B) $10 \mathrm{m} / \mathrm{s}$
(C) $30 \mathrm{m} / \mathrm{s}$
(D) $60 \mathrm{m} / \mathrm{s}$

Answer 1

Hint: We know that mechanics is the area of physics concerned with the motions of macroscopic objects. Forces applied to objects result in displacements, or changes of an object's position relative to its environment. Keeping this in mind, we can solve the given question.

Complete step by step answer
 We know from the data given in the question that,
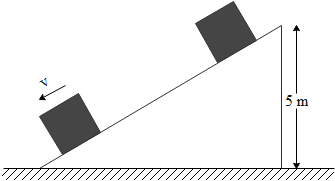
The height $\mathrm{h}=5 \mathrm{m}$
The gravitational acceleration $\mathrm{g}=10 \mathrm{ms}^{-2}$
Using conservation of energy, we get that,
$\mathrm{mgh}=0.5 \times \mathrm{mv}^{2}$
Cancelling mass from either side of the equation, we get
$10 \times 5=0.5 \times \mathrm{v}^{2}$
$\mathrm{v}=\sqrt{100}=10 \mathrm{m} / \mathrm{s}$

Therefore, the correct answer is Option B.

Note: We must effectively be able to draw free body diagrams. Free-body diagrams are diagrams used to show the relative magnitude and direction of all forces acting upon an object in a given situation. A free-body diagram is a special example of the vector diagrams.