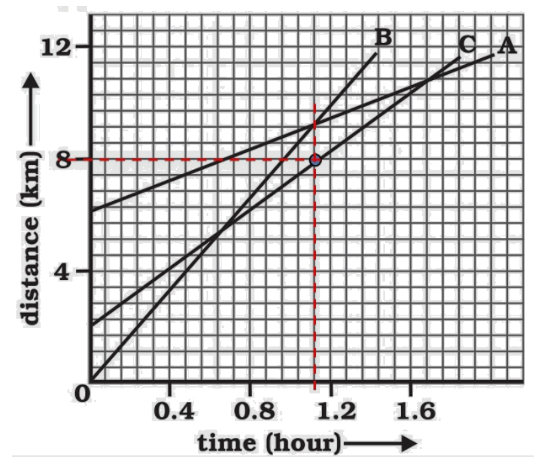
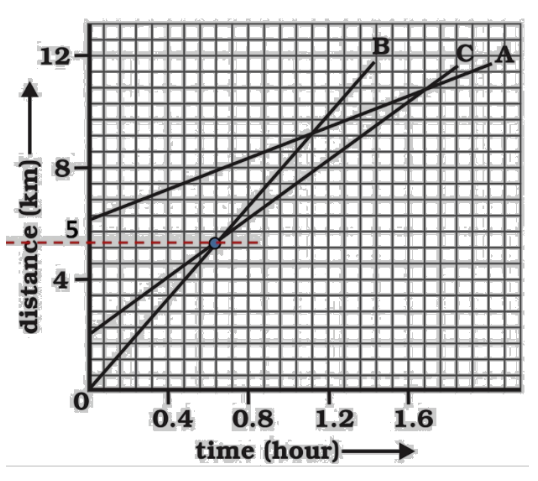
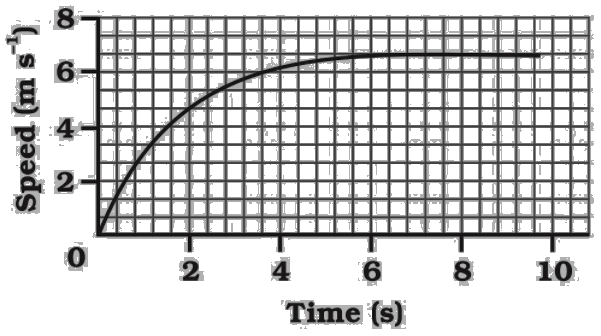
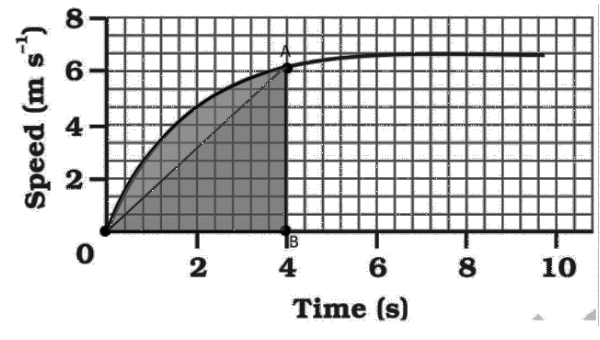
NCERT Solutions for Class 9 Science Chapter 7 - Motion FREE PDF Download
NCERT Solutions for Class 9 Science Chapter 7 Motion
FAQs on NCERT Solutions for Class 9 Science Chapter 7 Motion
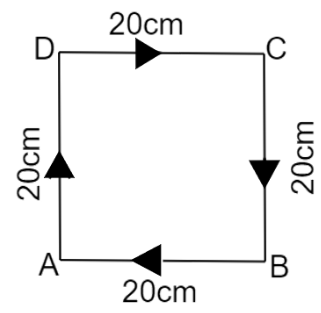
1. In regard to the NCERT Solutions Motion Class 9, Can an object have zero displacements if it has moved through a distance? If yes, then support your answer with an example or proof.
Yes, an object can move a certain distance without having any displacement. The logic behind this statement is that the displacement is defined as the shortest distance between the final and initial position of the object.
This means that even if an object moves through a large distance, it should come back to its initial position. In that case, the corresponding displacement of the object will be zero. Students can refer to class 9 science chapter 7 to learn more.
2. Mention the condition under which the magnitude of the average velocity of an object can be equal to the value of average speed, explained in class 9 science chapter 7 question answer.
We know that the average speed is the total distance that is travelled in a time frame and velocity is the total displacement in the time frame. The magnitude of average velocity and average speed will be the same when the total distance that is travelled is equal in its value to the displacement.
3. What I will learn from NCERT solutions for class 9 science chapter 7 motion exercise?
Chapter 7 of Class 9 Science is “Motion”. Students can learn about motion, its examples, types of motion including translational motion, rotational motion, linear motion, periodic motion, simple harmonic motion, projectile motion and oscillatory motion, and the laws of motion, from the NCERT Solutions for Chapter 7 of Class 9 Science. They can refer to the solutions to easily understand the concepts, especially if they have any confusion.
4. How can I solve the problems from the NCERT Solutions for Class 9 Science Chapter 7 class 9 motion effortlessly?
Students should try to solve all the questions and numerical problems of Chapter 7 of the Class 9 Science NCERT textbook. If they get stuck on any question or have a doubt about any topic, they can refer to the NCERT Solutions for Class 9 Science Chapter 7. The answers to all questions from the NCERT textbook of Class 9 Science are provided in the NCERT Solutions.
5. Is it important to study NCERT Solutions for motion class 9 questions answers?
Yes, ut is very important for the students to study NCERT solution of every chapter to score good marks in CBSE Class 9 exam. By studying the NCERT study material they will exactly know from which portion to study so that they can perform well in their exam.
6. What is motion and the types of motion in Class 9 motion NCERT Solutions?
Any change in the position of an object is defined as motion. There are various types of motion, including translational motion, rotational motion, linear motion (rectilinear motion and curvilinear motion), periodic motion, simple harmonic motion, projectile motion, and oscillatory motion. This is because the motion of an object is directly related to the type of force acting on the body.
7. How to download the NCERT Solutions for class 9 chapter 7 science?
Students can download it by clicking on NCERT Solutions for class 9 science chapter 7 exercise question answer. On this link, you will find the NCERT Solutions for Chapter 7 of Class 9 Science provided by Vedantu free of cost. You can also find the NCERT Solutions for all chapters of Class 9 Science as well as other subjects such as Maths, English, Hindi, and Social Science. All these can be downloaded from the Vedantu app also. Several other study materials such as important questions, revision notes and previous year question papers are also available on Vedantu (vedantu.com).
8. What are the different types of motion described in the class 9 science ch 7?
The different types of motions are:
Uniform
Non- Uniform
9. How can we present motions with graphs?
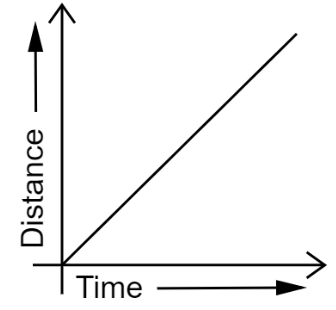
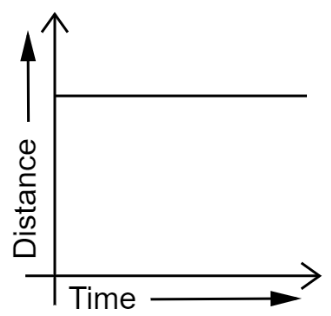
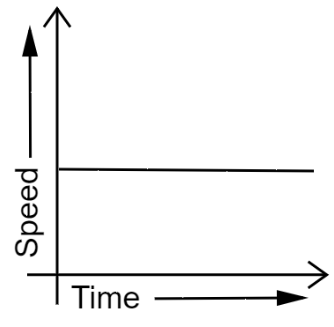
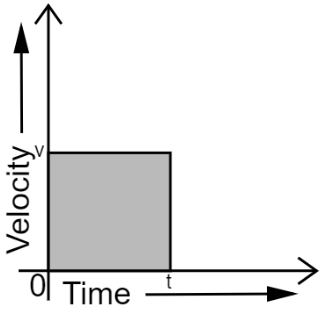
We can represent motions by distance-time graph and velocity-time graph. Students can download and refer to motion class 9 NCERT solutions to clear the doubts related to the chapter.
10. What is the difference between speed and velocity described in class 9 science chapter 7 question answer?
Feature | Speed | Velocity |
Type of Quantity | Scalar | Vector |
Information Provided | Magnitude (how fast) | Magnitude (speed) and Direction |
Example | 60 kilometres per hour | 60 kilometres per hour towards the north |




















 Watch Video
Watch Video